Tuesday, October 28, 2008

derivation of mean and variance of binomial distribution

derive the mean and variance of the binomial distribution
now to find the variance, we rewrite x^2 as x(x-1) +x before we start out



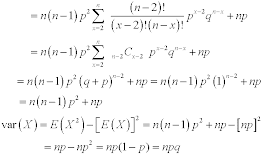
for explanation of mean of poisson distribution try the link mean of poisson distribution

for explanation of mean and variance of discrete uniform distribution try the link mean of the discrete uniform distribution



disclaimer:
There is no guarantee about the data/information on this site. You use the data/information at your own risk. You use the advertisements displayed on this page at your own risk.We are not responsible for the content of external internet sites. Some of the links may not work





13 comments:

  1. AnonymousJune 11, 2009 at 2:27 AM

    thank u....

    ReplyDelete
  2. AnonymousSeptember 19, 2009 at 12:51 PM

    thanx man .... d derivation n my book ended in just 2 lines !!!

    ReplyDelete
  3. AnonymousApril 28, 2010 at 4:25 AM

    thanx man .... d derivation n my book ended in just 2 lines !!!

    ReplyDelete
  4. EricaJuly 18, 2010 at 11:08 AM

    thanks :)

    ReplyDelete
  5. AnonymousJanuary 12, 2011 at 9:16 AM

    thanks dude..............

    ReplyDelete
  6. AnonymousJanuary 29, 2011 at 11:37 PM

    Thank you!! This is just what I was looking for!

    ReplyDelete
  7. AnonymousMay 19, 2011 at 10:05 AM

    THANKS!

    ReplyDelete
  8. CharyMay 25, 2011 at 2:41 PM

    No matter when you posted, its still helpful to me, thanks. I understood everything as the steps are easy to follow.

    ReplyDelete
  9. AnonymousSeptember 1, 2011 at 11:24 AM

    Thanks Alot.. As A B.Tech 2nd Year Student it helps me alot!

    ReplyDelete
  10. AnonymousSeptember 21, 2011 at 2:57 AM

    Thank u vry much...

    ReplyDelete
  11. AnonymousMarch 9, 2012 at 6:25 AM

    thank you !

    ReplyDelete
  12. SAMARJIT SAHASeptember 22, 2012 at 12:53 PM

    Thank u so much

    ReplyDelete
  13. AnonymousNovember 19, 2012 at 5:08 AM

    Deviation from mean x-μ
    Square deviation from mean Sum of the squares of deviation from mean=∑▒〖(x-μ)〗^2
    The mean sum of the squares of deviation from the mean (μ) is=1/n ∑▒〖(x-μ)〗^2
    The root mean square deviation is = sqrt(1/n ∑▒〖(x-μ)〗^2 )



    The standard deviation is nothing but the root mean square deviation .

    ReplyDelete

please leave your comments