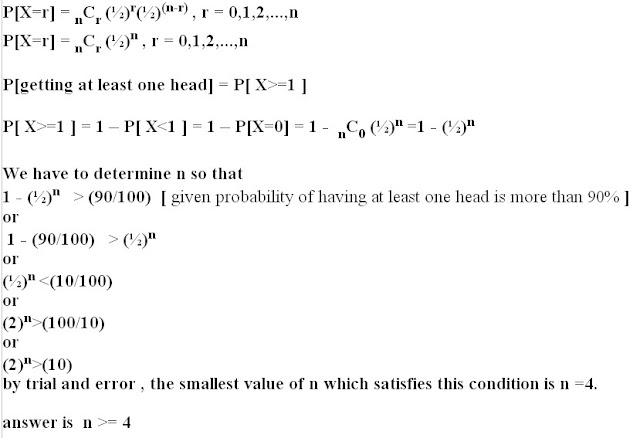
finding n of binomial distribution given the probability from miscellaneous 12th cbse ncert mathematics
How
many times must a man toss a fair coin so that the probability of
having at least one head is more than 90%?
Let
n be the required number of times the man must toss a fair coin so
that the probability of having at least one head is more than 90%
Let
X be the number of heads obtained when the coin is tossed n times.
Assuming
X follows binomial distribution with
n=n
p
= (½)
q
= 1 – p = (½)
P[X=r]
= nCr prq(n-r) , r =
0,1,2,...,n
disclaimer
There
is no guarantee about the data/information on this site. You use the
data/information at your own risk. You use the advertisements
displayed on this page at your own risk.We are not responsible for
the content of external internet sites. Some of the links may not
work
link to index of other miscellaneous problems on probability of cbse ncert 12th mathematics
index of more problems on baye's theorem for ncert cbse mathematics
link to index of other miscellaneous problems on probability of cbse ncert 12th mathematics
index of more problems on baye's theorem for ncert cbse mathematics
No comments:
Post a Comment
please leave your comments